Lu yudong^1,2) Li peicheng¹⁾

1)College of environmental sciences and engineering ,Chang’an University, Xi’an 710054,China；

2) College of resources and environmental sceiences, Chongqing University, 400044, China

 

Abstract: There are stagnant flow in confined aquifer during pumping test. In this paper, inertial equation is derived by applying contiguous media dynamics theory. Ground water transport equation, which contain acceleration term, is a attenuation wave equation, and include time parameter representing by . The spread velocity of water head is function of catchment rate, and water and solid media is more compressive , the spread velocity is more little. In fact ,there is a lag time between the water level change of well in confined aquifer and its observation well. That lag time is depended on character of aquifer and observation distance.

Keywords: Confined aquifer; Stagnant flow; water head spread; Wave equation

 

 

Meizer is the first to realize the compressibility of confined aquifer^[1]. Traditionally, the unstable water level change of well in confined aquifer was based on the instantaneous spread of water head. But , generally, in the pumping test we can observe that the change of the water level in the pumping well does not instantly lead to that of the observation well, that is to say, there is a time span or lag time for the process of the spread of water level in seepage field. That lag time has something to do with the character of aquifer, the character of water and the distance of water heads. Based on the infiltrative damping theory^[2][3], porous wall can be regarded as the obstacle to linear movement of sticky liquid. In fact, just because of the block effect of media^[4-6], the spread of water head gets a certain velocity. Muskat believes that the velocity of confined aquifer is similar to that of sound^[7]. Meinzer agrees with Muskat on that point and believes that quantitative criterion of the velocity is related to the level of measurement. Owing to the condensability of solid media and water media, there lies a process of attenuation and adjustment to water pressure in media, which can be called lag time. In this paper, inertial equation is derived by applying contiguous media dynamics theory; parameter of the spread of water head is obtained and meanwhile its physical significance is stated.

1       The Inertial Transport Equation

We base our seepage flow research on representative elementary volume（REV）, suppose single composition water and porous solid media as continuous media which permeate the whole space. From this point of view, we generally believe that the relationship between seepage velocity and specific capacity is like this^[2][3][8]:

（1）

in this equation, ——specific capacity，dimension LT^－1；n ——porosity，without dimension；——seepage velocity，dimension : LT^－1. formula （1）is sometimes called Dupuit－Fochheimer equation.

Suppose f(x,t) as a certain physical quantity, such as mass, density, momentum and energy .

is the system density, n is the system hole distribution ratio, U（t）is the volume of system at the time of t (fig.1), then from Renold transport equation, we can get the equation,

（2）

in this eqution, is the volume of controlling body; is seepage; is system volume；and represents the direction of the normal line outside of control volume.

 

 

Fig.1 The system and control volume of a aquifer

 

For

（3）

and since , taking differential coefficient calculation, and together with (1), (2) we can get

（4）

and (4) is the continuous equation of ground water seepage.

In order to get the transport equation, take a random entity whose mass is m, change its volume but keep its mass , then we get the momentum of this entity,

（5）

taking differential coefficient calculation on (5), and together with (2) , we can get

（6）

by Gauss law, taking (4) and (1) into consideration, we can get,

▽ （7）

agrees with the composition of forces on , including surface force，entity force, and infiltration resistance.

The expression of surface force is,

here, ,which is a tensor, is the direction of outside normal line.

Using gauss law on this equation, we get,

In the traditional hydrodynamics only surface force and entity force are taken into consideration. is related to the fluidity of liquid and changes with pressure , temperature and so on. If water is static in porous media, the value of is 0. Another example, when ，.

Thus, we can get a movement equation:

▽ （8）

In Cartesian Coordinates, make the up direction of z positive, suppose is acceleration of gravity, then the inner entity force of is , unit entity force is . Surface force is the pressure from nearby representative elementary volume, so force can only act on the controlling body , surface force ▽.

Finally, suppose infiltration resistance and        in ground water seeping field takes on linear relationship(agrees with linear law). Its proportion coefficient is ；is dynamic viscous coefficient, dimension : ML^－1T^－1；is infiltration rate, dimension L²; thus the infiltration resistance for volume unit is:

(9)

then, (8) can be turned into(10)

▽▽（10）

in Darcy law, suppose the area of application is laminar flow state and the item of inertia is not considered, and are both constant. For , , thus 。（10）is turned into

▽ （11）

And (11) is namely 达西 equation^[9]. It is an approximate law in certain special condition.

In (9), the first item local acceleration is much larger than the second item transport acceleration. In common cases, the transport acceleration can be ignored; thus we get the linear transport equation which includes the item local acceleration:

▽ （12）

using water head, it can be expressed like this,

▽ （13）

in this equation, ；is infiltration rate，dimension L；is dynamic viscous coefficient, its dimension is ML^－1T^－1.

2       The Spread of Water Head in Confined Aquifer

The traditional Darcy equation is a linear diffuse equation. Water head spreads at unlimited velocity, i.e. the changes of water level can be spread everywhere in the infiltration field. Actually, there is a lag time between the water level changes of well in confined aquifer and that of its observation well and that lag time is depended on character of aquifer and the observation distance.

a)        Physical significance of underground water movement equation

The underground water movement equation, which includes the acceleration item, is a damped equation which includes the time unit . In common cases, water density and viscous coefficient are the function of time and pressure, but their changes are little under normal pressure and temperature. Here we suppose,

（14）

, the first item in equation (13), shows the runoff changes resulted from the unsteadiness of flow field. It is similar to the lag time equation of heat exchange in isotropy media, which is discovered by Cattaneo and Vernotte in their thermodynamics analysis research^[9][10]. The dimension ofis T, it has a time unit which stands for the lag time.

b)       Continuous equation expressed by water head

and are function of compression coefficient in porous media（dimension NL²）and compression coefficient （dimension NL²）in solid media. Their changes can be shown like this,

（15a）

in this equation, is the pressure of static water, and its dimension is NL².

（15b）

Taking (15) into (14) with substitution method, in view of ▽, and therefore we get,

▽ （16）

When matter and temperature are kept unchanged in compressive flow field, , and its underground water head is,

（17）

Drawn from (16) and (17), we get (18)

（18）

here, , is the catchment rate in aquifer, its dimension L^－1.

Take (13) into (17) with substitution method, and omit the quadratic differential coefficient of H, and we can get the continuous equation expressed with water head,

△ （19）

△，is Laplacian operator.

3 Lag effect of Underground Water Head

Equation (19) is continuous equation of underground water seepage with inertia in it, and it’s a damped wave equation^[11][12], different from traditional heat exchange equation. According to the physical significance of wave equation, the wave velocity or the spread velocity of water head in seeping field can be drawn,

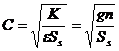（20）

water head spread velocity is mainly the function of . The greater the compressibility of water and solid media is, the lower the spread velocity of water head is. Generally speaking, in confined aquifer from fine sand to gravel, water head spread velocity C is 41－185m/s, which is lower than the velocity of sound.; correspondingly, water head spread lag time is quite little, less than one second.

4 Conclusion

The character of water head spread in confined aquifer is related to the solid media and water media in aquifer, and its spread velocity is 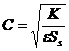. Generally speaking, the thicker the granule is, the higher the spread velocity of water head is, vice versa. The well flow movement equation, taking water head spread into consideration, which is different from Theis model, should contain the item of water head spread parameter. When the lag time is quite short, it can be turned into the traditional confined aquifer Theis model. Therefore, in lower penetrability aquifer containing mainly fine sand, the spread velocity of water head is lower, and the lag time is longer. Taking water head lag effect into account, the well flow equation is much similar to the conditions of the real well flow.

In a serious hydro-activities such as water resource development and management, it’s quite important to know the distribution of hydraulic pressure field in underground water movement and its spatial changes. Taking water head spread lag effect into consideration in hydro-geology model will no doubt make the model more perfect to be more similar to the conditions of the distribution of real pressure in seepage field and reduce the calculation error at the beginning of the pumping test, and what’s more important is that during a certain period of time, it can simplify the border conditions, that is to say, the area outside of the water head spread border has no effect on each physical quantity(such as pressure, density, runoff and so on). In this way, we can greatly reduce the work of calculation and improve the precision of calculation.