M. Reza Ghanbarpour¹，Shabanali Gholami²，Elham Ahmadi³，Mehdi Teimouri⁴

1. Faculty of Natural Resources, University of Mazandaran, P.O.Box: 737, Sari, Iran. Tel: +98 151 422 2984-5, Fax: +98 151 422 2982, email: m_ghanbarpour@yahoo.com.

2. Soil Conservation and Watershed Management Research Institute, Tehran, Iran.

3. Regional and urban planning, Tehran, Iran

4. Faculty of Natural Resources, University of Mazandaran, Sari, Iran

Abstract:Estimating the amount of groundwater contributions to stream flow within a watershed is a critical aspect of many hydrogeologic investigations. Determination of this variable could be very challengeable due to uncertainty involving separation of different parts of stream flow. The base flow in most watersheds can be assumed as the water discharged from groundwater aquifers. In this study base flow separation and regional analysis of effective variables were used to evaluate the spatial variability of groundwater contributions. Recursive digital filter with filter parameter of 0.925 were used to separate daily stream flow data into direct runoff and base flow, which has been shown the most accurate base flow separation methods in previous studies. Karun watershed in south west of Iran was selected for this study. Stepwise multiple regression analysis was used to extract regional relationship to estimate base flow index (BFI) at the ungauged watersheds. Seven watersheds with different characteristics were used to evaluate spatial variability of BFI through the region. Possible applications of the results of this research in the study area were discussed.

Key words: Groundwater, base flow, digital filter, regionalization.

Introduction

Determination of groundwater discharge is crucial for efficient water resources development as well as for water pollution management at the watershed scale. The total stream flow can be viewed as consisting of mostly two parts, direct runoff and baseflow. The direct runoff is the storm runoff that results from rainfall excess. The baseflow is the water discharged from groundwater aquifers. Estimating the monthly and annual volume of groundwater contributions to stream flow within a watershed is a critical aspect of many hydrogeologic investigations including water supply, irrigation planning, wastewater dilution, navigation, hydropower generation and aquifer recharge and characterization (Brutsaert & Nieber, 1977, Troch et al. 1993, Dingman, 1994, Szilagyi, 2004). Baseflow, which is a major component of streamflow, can be considered a reasonable approximation of groundwater discharge to a stream (White & Sloto, 1990, Holtschlag & Nicholas, 1998, Smakhtin, 2001). This is particularly useful for

numerical groundwater-flow modeling researches. Some researchers (Hoos, 1990, Mau & Winter, 1997, Rutledge & Daniel, 1994) have shown that baseflow can be used to derive basin average estimates of groundwater recharge. In addition, an understanding of the outflow process from groundwater is also essential in water budgets and catchment’s response analysis. Numerous analytical techniques have been developed to separate base flow from total stream flow (McCuen, 1998; Nathan and McMahon, 1990). Ghanbarpour, et al. (2006) has shown that the recursive digital filter base flow separation technique with the filter parameter of 0.925 has the most accurate results in south west of Iran. In this study recursive digital filter as an automated base flow separation technique with the filter parameter of 0.925 were used to estimate daily baseflow data series in seven watersheds in Karun basin in south west of Iran. Then, groundwater contributions to total stream flow as BFI were estimated in all watersheds under study.

To study the regional pattern of BFI and contribution of groundwater to stream flow, relationship between several physiographic variables and the BFI were developed using stepwise multiple regression analysis.

The overall objective of this research is to gain a better understanding of the contribution of groundwater component of stream flow at different spatial scales. The most accurate regional model could be used to estimate the BFI of all hydrological units, which have not data observation. This study is intended as an important step toward improving the knowledge of the temporal and spatial baseflow characteristics of the basins of area, which is crucial to prevent groundwater depletion and pollution and to define a rule for establishment of conjunctive use in the region.

Methods and Aterials Study Area

The Karun basin studied in this project is located in the southwest of Iran from 30º 16´ to 32º 39´ N latitude and 49º 33´ to 52º 0´ E longitude (Fig 1). The basin covers an area of approximately 24,141 square kilometers, with elevations between 800 and 4400 m. The mean annual precipitation of the area is about 650 mm. The Poleshaloo hydrometric station, at the outlet of the basin with an elevation of 800 m, has recorded an average annual runoff of 341.9 m³ s^-1. The low flow season generally begins in July and ends in November. In this research, six basins with different characteristics in the study area are investigated. Details of the basins under study including average annual runoff, area, and the elevations of hydrometric stations, are listed in Table 1. The runoff data employed in this study consist of daily flows, in cubic meter per second at seven watersheds depicted in Figure 1, in a period of thirty years from 1969 to 1998. The data have gathered from Water Resources Research Organization (TAMAB Co.) in Iran.

Figure 1. Location of the study area in Iran

Table 1. Details of the sub-basins under study

Base FlowIndex (BFI)

BFI is a dimensionless ratio, which developed by Lvovich (1979) and the Institute of Hydrology (1980). BFI can then be calculated as the volume of base flow divided by the volume of total runoff for each year or for total period of record. This index can present some information about the proportion of the stream flow that originates from stored sources. The index can be calculated from stream flow data or estimated from basin geology (Institute of Hydrology, 1980). The first step in estimation of BFI is separating of base flow from total stream flow. To determine BFI in watershed under study base flow separation methods can be used. Base flow separation techniques divide a stream hydrograph into two major components: base flow and direct runoff. In this research recursive digital filter with the parameter value of 0.925 was used for separating of base flow from total stream flow. Nathan & McMahon (1990) applied recursive digital filter as an automated approach for partitioning stream flow into base flow and direct runoff. Recursive digital filter can be written as:

      (1)

where is the filtered quick response at the kth sampling instant, is the original stream flow, and is the filter parameter and is the filtered base flow. The filter is passed over the data three times. The parameter affects the degree of attenuation, and the number of passes determines the degree of smoothing. Nathan & McMahon (1990) found that the value of the filter parameter that yielded the most acceptable base flow separation was in the range of 0.9-0.95. They found that the simple digital filter with a parameter set to 0.925 produced more accurate yields and repeatable results compared to the simple smoothing and separation rules. They found that the results were comparable to those obtained by using traditional graphical techniques. Ghanbarpour et al. (2006) has shown that in Karun basin filter parameter of 0.925 will has the most accurate results. The results of that reseach were gained by using the comparison of the base flow separation methods with recession analysis as a most reliable but at the same time very time consuming technique. Although the recession analysis is very accurate but this method is almost impractical and tedious when it should be applied to a large number of basins furthermore they cannot be formulated. Figure 2 shows an example of the graphical output of base flow separation for Pole Shaloo hydrometric station using the recursive digital filter method for the water year of 1979-80.

BFI has many practical applications, especially in rainfall-runoff modeling. Also it can be used as a basin characteristic to compare the flow characteristic of different basins. In this research the BFI values for fourteen basins using the selected baseflow separation method were calculated.

Regionalization of BFI

To study the regional pattern of BFI and contribution of groundwater to stream flow, relationship between several hydrologic and physiographic variables and the BFI were developed using stepwise multiple regression analysis. As lacey and Grayson (1998) stated, base flow is a function of a large number of variables that include topographic, climatic and etc. Nathan et al. (1996) carried out a regression analysis for base flow index in terms of a number of catchment properties for 164 catchments in the state of Victoria, Australia. The most accurate regional model could be used to estimate the BFI of all hydrological units, which have not data observation.

Results and discussion

In this research recursive digital filter with the filter parameters of 0.925 were used to estimate base flow data series in daily and monthly time scale in seven watersheds in south west of Iran. Figure 2 shows an example of the graphical output of baseflow separation for Pole Shaloo hydrometric station using the recursive digital filter method the water year of 1979-80.

In this research, the monthly and annual BFI data series for all seven sub-basins were estimated using recursive digital filter method with parameter value of 0.925. Figure 3 shows summary statistics of mean, minimum and maximum annual BFI values for watershed under study. As can be seen in Figure 3, annual average of BFI varies from 0.79 to 0.88. It is evident that more than 80 percent of the annual stream flow could be considered as baseflow or groundwater discharge in the study area and similar basins.

To study the regional pattern of BFI and contribution of groundwater to stream flow, relationship between several hydrologic and physiographic variables and the BFI were developed using stepwise multiple regression analysis. Base flow is a function of a large number of variables. Therefore in this study some topographic and hydro-climatic variables were considered to extract the regional relationship for estimation of BFI. Area, weighted average of elevation, annual runoff, and snowmelt runoff is the most important variables that we considered for this research. The results of stepwise regressions using different sets of variables have shown in Table 2. Significance percent of the regional relationships were shown in Table 3. The regression analysis based on Table 2 and 3, indicated that the best correlation was between BFI and the area of watersheds in square kilometer. This relationship is significant at the 99% confidence level and is equal to 0.685:

(2)

Where:

is base flow index (%),

is watershed area (km²).

Eq. (2) could be used to estimate the average annual BFI in all hydrological units in the study area, which are ungauged. Figure 4 has shown the relationship between BFI and Area in study. As can be seen in Table 2 and 3, there is a significant relationship between BFI and area and annual runoff for the watersheds under study. On the other hand there are unsignificant relationship between snowmelt runoff and elevation with BFI. As can be seen in Table 2., when more than one variable is added to the equations, will increased. On the other hand significance of the equation and also incorrelation of the independent variables produce unsuitable models, regarding to estimation of BFI for unguaged watershed in study area.

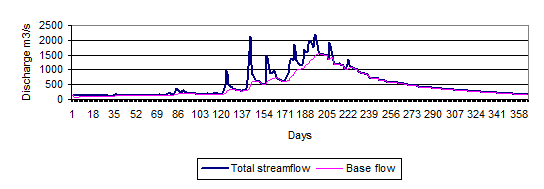
Figure 2. base flow separation results at the Pole Shaloo hydrometric station for the water year of 1979-80, based on recursive digital filter with the parameter value of 0.925.

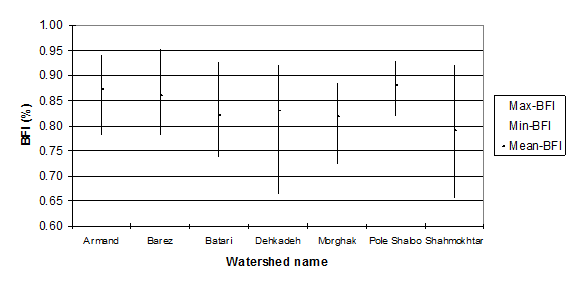                                       Figure3. Variability of BFI (mean, Maximum and minimum) values in study area.

Table 2. regional models for estimation of BFI in study area.

is base flow index (%), is watershed area (km²), is average annual runoff (m³ s^-1), H is weighted average elevation of whole watershed (m), SWE is annual estimated snowmelt runoff (mm).

Table 3. regional models for estimation of BFI in study area.

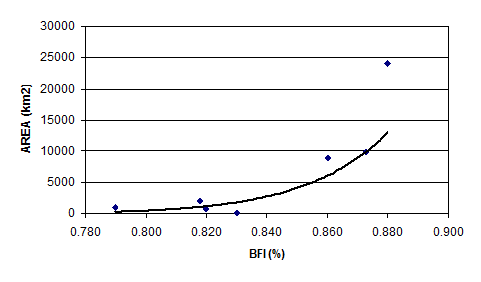
Fig 4. Relationship between BFI and Area in study area

Conclusion

Estimating the contribution of groundwater recharge is an important aspect of many hydro-geologic and water resources investigations, especially numerical modeling and water budget analysis, at the watershed scale. In the case of water quality availability, base flow estimates can be integrated with this information to estimate groundwater loading. As an important application, groundwater discharge could be used in conjunction with land use change trends to investigate the effects changes in land management systems at the watershed scale, which is a critical aspect in water resources planning and management.

In this research, an automated base flow separation technique, recursive digital filter was used to determine BFI values for seven watersheds in south west of Iran. Application of automated recursive digital filter is easy, accurate, consistence and does not need subjective efforts, which will assure applicability of the base flow values as hydrological variables for future water resources management during low flow season and other applications.

Regional analysis of BFI in the area has shown that the most accurate relationship is exists between area and BFI in all watersheds under study. Because of intercorrelation and low significance of the multiple regressions equations, only model number 2 was selected as the most accurate model for regional estimation of the BFI at the unguaged watersheds in study area.

This research has shown that there is a significant dependency of groundwater contribution with watershed area. As can be seen in Figure3, BFI values in large watersheds are more in comparison with small watersheds. On the other hand, there are more differences between maximum and minimum of BFI values in small watershed, such as Dehkadeh and Shahmokhtar. Whereas, more variabilities of BFI can be seen in large watersheds such as Pole shaloo and Armand.

The results of this study could be used to define contribution of groundwater and base flow data series for ungauged basins in the region using regionalization procedures regarding to importance of low flow seasons with viewpoints of water quality and environmental consequences of drought periods. On average, groundwater discharge represented approximately more than 80% of total annual streamflow for the stations modeled. This highlights the importance of managing surface water and groundwater as a single interconnected resource.